Random walk with weight in 2 dimensions

[Kevser_Cifci]

Hello!
I'm trying to code a random walk with the weight function :W(k)=k^alpha.
The probability to choose the next position is :

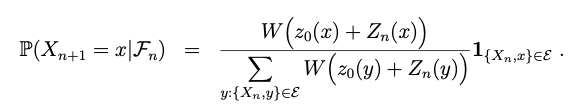
W is the weight function defined earlier.
z0 is the initial weight of the vertex/position (in the code I choose z0 = 1)
Zn(x) is the number of time the vertex x has been visited.

Here you can see what I've tried to do:

Next_step = function(i,j,W){
  
  l = nrow(W)
  prob = rep(NA,4)
  
  prob[1] = W[i+1,j]
  prob[2] = W[i,j+1]
  prob[3] = W[i,j-1]
  prob[4] = W[i-1,j]

  next_step = sample(1:4, 1,replace = TRUE, prob = prob)
  
  
  if (next_step == 1) { # down
    i = i+1
  } else if (next_step == 2) {  # right
    j = j+1
  } else if (next_step == 3) { # left
    j= j-1
  } else if (next_step == 4) { # up
    i = i-1
  }
  return(c(i,j))
}
RW_2d_start = function(n,pas, alpha){
  
  Grille = matrix(1, nrow = n+2, ncol = n+2)
  Grille[c(1,n+2),] = 0
  Grille[,c(1,n+2)]=0
  W = Grille
  
  axe_x = rep(NA,pas)
  axe_y = rep(NA,pas)
  d = rep(NA,2)
  
  if (n%%2 != 0) {axe_x[1] = floor(n/2)} else {axe_x[1] = n/2} ## middle
  axe_y[1] = axe_x[1]
  Grille[axe_x[1],axe_y[1]] = Grille[axe_x[1],axe_y[1]]+1
  W[axe_x[1],axe_y[1]]=Grille[axe_x[1],axe_y[1]]^alpha
  for (i in 2:pas){ 
    d[1:2]= Next_step(axe_x[i-1],axe_y[i-1],Grille) 
    axe_x[i]=d[1] 
    axe_y[i]=d[2] 
    Grille[d[1],d[2]] = Grille[d[1],d[2]]+1
    W[d[1],d[2]]=Grille[d[1],d[2]]^alpha
  }
  return(list(path = data.frame(step = seq_along(axe_x), x = axe_x, y = axe_y),
              Grille = Grille))
}

N = 100
pas = 5000
alpha = 1
res <- RW_2d_start(N,pas,alpha)
res_path <- res$path
plot1 <- ggplot(res_path) +
  theme_classic() +
  geom_path(aes(x = x, y = y),) +
  labs(
    title    = "alpha",
    x        = "x position",
    y        = "y position") 
plot1

Does anyone know if this is correct?

Thank you !