Thanks for your answers,
So, i will try to explain with my weak english level ^^'
dCanada = diff(Canada,1)
PACF(dCanada)
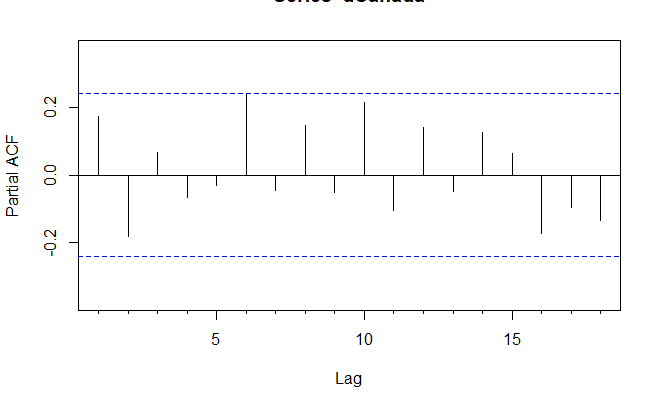
I diff my serie then i made PACF : i found a weak autocorrelation at order 6
EACF(dCanada)
Box.test(dCanada, lag=12 , type="Ljung-Box")
AR/MA
0 1 2 3 4 5 6 7 8 9 10 11 12 13
0 o o o o o o o o o o o o o o
1 x o o o o o o o o o o o o o
2 x x o o o o o o o o o o o o
3 x o o o o o o o o o o o o o
4 x o o x o o o o o o o o o o
5 o o o x o x o o o o o o o o
6 o x o o o o o o o o o o o o
7 x x o o o o o o o o o o o o
Box-Ljung test
data: dCanada
X-squared = 10.216, df = 12, p-value = 0.597
**So , i made EACF and found a ARMA(0,0) .... really weird... **
Moreover, LB test at lag 12 have p-value > 0.05 ......
auto.arima(dCanada, trace=TRUE, ic=c("aic"))
ARIMA(2,0,2) with non-zero mean : Inf
ARIMA(0,0,0) with non-zero mean : 175.2989
ARIMA(1,0,0) with non-zero mean : 175.3013
ARIMA(0,0,1) with non-zero mean : 174.2514
ARIMA(0,0,0) with zero mean : 173.3635
ARIMA(1,0,1) with non-zero mean : 175.4723
Best model: ARIMA(0,0,0) with zero mean
Series: dCanada
ARIMA(0,0,0) with zero mean
sigma^2 estimated as 0.7855: log likelihood=-85.68
AIC=173.36 AICc=173.43 BIC=175.55
So, i decide to make an auto.arima et found ARIMA(0,0,0) with non mean as best model....
But i wasn't convinced by this model because i founded autocorrelation with PACF...
So i test a lot of model and found ARMA(2,2) with a better AIC than the ARMA(0,0) ...
ARMA0_0 = Arima(dCanada, order = c(0,0,0), include.mean=FALSE)
ARMA2_2 = Arima(dCanada, order = c(2,0,2), include.mean=FALSE)
coeftest(ARMA2_2)
AIC(ARMA2_2)
AIC(ARMA0_0)
z test of coefficients:
Estimate Std. Error z value Pr(>|z|)
ar1 -1.460105 0.114566 -12.7447 < 2.2e-16 ***
ar2 -0.493069 0.113722 -4.3357 1.453e-05 ***
ma1 1.944239 0.068811 28.2549 < 2.2e-16 ***
ma2 0.999974 0.069793 14.3278 < 2.2e-16 ***
---
Signif. codes: 0 ‘***’ 0.001 ‘**’ 0.01 ‘*’ 0.05 ‘.’ 0.1 ‘ ’ 1
[1] 166.2296
[1] 173.3635
My forecast seems to be very good with the ARMA(2,2) and all white noise hypothesis IS OK with it.
How can i be sure ARMA(2,2) is the best model ? Why auto.arima don't give me the ARIMA(2,0,2) model directly ?
Can i found better with an AR(6) ? Or MA(6) ?
Thanks,
Paul
